Schematically, the objective of this research line is the design of advanced systems control methods, as well as their application to the dynamics of biological systems and in particular to the control of epidemic systems. The result of the adaptation of the theory of automatic control to epidemic control constitutes a relevant contribution to the serious problem posed by infectious diseases at a health, productive and economic level and directly framed in the RIS3 priorities. In this sense, the specific objectives of this line are:

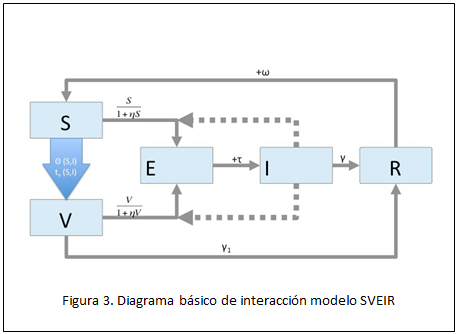
Development of advanced systems control schemes. Adaptation to biological models of an epidemic nature. Design of robust control schemes (SMC), predictive (MPC), intelligent (neuro-fuzzy-ANFIS) and combination thereof, formal theoretical development and stability analysis. Development of new deterministic epidemic models from the basic SIR / SEIR by incorporation of forcing terms of noise type of Wiener. This type of noise is of particular interest for differential equations since it is an integral stochastic process of Gaussian white noise with independent increments so that the process as a whole is independent of its previous history. The Wiener processes, or Brownian movements, are relevant in economic models, physics, mathematical theory of finance, stochastic calculation, diffusion processes, theory of potentials, errors of instruments in filtering and control theory.

Application to impulsive vaccination schemes. This second objective, which naturally extends objective 1, consists of studying the dynamic system of the sub-populations in the case of disturbance of equilibrium when the impulsive vaccination campaigns are not regular neither in the fraction of vaccinated population nor in the inter-vaccination time period. The first can be interpreted in the context of control theory as a variable gain dependent on the "gain-scheduling" state and the second as a state-dependent adaptive sampling period. The main novelty is the design of vaccination laws for these new models. The team has previous experience in impulsive systems and an older experience in adaptable sampling for adaptation of signals, subject of doctoral thesis of the principal investigator.

Treatment of discrete epidemic models and their control by vaccination laws. Design of ad-hoc control strategies through vaccination to stop the spread of epidemics in a population using modeling techniques based on the linearization of non-linear models "exact feedback input-output linearization approach". The models are to be obtained from the discretization of continuous SEIR and SIR models, both in the deterministic version and in the stochastic version similar to that of objective 1. The control strategies to be developed will be based on vaccination control laws synthesized using the technique of feedback of state variables to obtain a linear controlled model in its input-output relationship. Basically, what is intended is to linearize the model in closed loop so that the output is the infected population with symptoms, while decreasing the order of the system in one when the total population is constant, and place the poles where you want , that is, prefix the own dynamics of the deterministic system in closed loop so that, the dynamics of the closed loop system is prefixed guaranteeing the global asymptotic stability in closed loop as well as other properties of the solution related to the relative stability and the positivity of the closed loop system. In this sense, it must be borne in mind that the epidemic models used for the study of the spread of infectious diseases are non-linear with non-linearities of the quadratic type and the eventual presence of delays. Parallel questions necessary to address are the study of the properties of stability, dimensioning of the solution (only in the case of non-standardized models) and positivity of the controlled model without or with the incorporation of an observer in the event that there is no precise information on all sub-populations of it. Note that, based on real-time information from the health authorities, it is easy to have information on the infectious sub-population in many types of epidemics but not in the other sub-populations. The fact of guaranteeing the property of positivity of the model is vital for the good formulation of the model in the real context since the nature of the problem requires that the temporal evolution of the partial populations does not pass through negative values. It is also intended to deepen the development of predictive techniques for vaccination in discrete variants.